Watch this video!
Exercise
1. Find the distance betwixt P(3, 2) in addition to Q(4, 3).
2. Find the distance betwixt (a, b) in addition to (a+b, a-b).
3. Which indicate is nearest to the origin, (2,3) or (-2,3)?
Saturday, January 18, 2020
Application Of Distance Formula
How volition yous demo that the given 4 points are the coordinates of vertices of a rectangle?
Watch this video!
Watch this video!
Area Of Triangle When Coordinates Of Vertices Are Given
Learn to honor the expanse of a triangle when the coordinates of its vertices are given.
Watch this video!
Watch this video!
Application Of Department Formula
Let us larn to notice the points of trisection using the Section Formula.
Watch this video!
Watch this video!
Application Of Department Formula
In what ratio a betoken divides the bring together of 2 given points?
We role the department formula...
Watch this video!
We role the department formula...
Watch this video!
11 Swell Mathematicians
Dated Magic Square
How to brand a Dated Magic Square?
1. In the offset row, write downwardly the appointment for which you lot desire to practise the Magic square.
3. Calculate the magic constant which is the amount of elements of each row, column, diagonal); past times summing upwards the elements of offset row. Magic constant =13+11+20+11 = 55. 4. Now, calculate the chemical cistron inwards row2, column 3 past times subtracting the other 3 elements of that sub-square by magic constant.
55 – (20+11+6) = 18.
5. Now, honour the blank box of column 3 past times subtracting the amount of other elements from magic constant. 55-(20+18+4) = 13.
6. Follow the same agency to consummate the row 2, column 2 chemical cistron from fundamental sub-square. 55-(18+13+5) =19
7. 2nd row 1st column volition survive 55 – (19 + xviii + 6) =12
4th row 2nd column volition survive 55 – (11 + xix + 5) = 20
8. 4th row 1st column volition survive 55 – (11 + xviii + 5) = 21 4th row 4th column volition survive 55 – (13 + xix + 13) = 10
9. 3rd row 1st column = 55 – (13 + 12 + 21) =9
3rd row 4th column = 55 – (11 + vi + 10) = 28
1. In the offset row, write downwardly the appointment for which you lot desire to practise the Magic square.
Remember to carve upwards the twelvemonth into two. I guide keep taken the appointment every 2d 13.11.2011.
2. Write whatsoever 3 consecutive numbers in the boxes every 2d shown below.
4. Now, calculate the chemical cistron inwards row2, column 3 past times subtracting the other 3 elements of that sub-square by magic constant.
55 – (20+11+6) = 18.
5. Now, honour the blank box of column 3 past times subtracting the amount of other elements from magic constant. 55-(20+18+4) = 13.
6. Follow the same agency to consummate the row 2, column 2 chemical cistron from fundamental sub-square. 55-(18+13+5) =19
7. 2nd row 1st column volition survive 55 – (19 + xviii + 6) =12
4th row 2nd column volition survive 55 – (11 + xix + 5) = 20
4th row 4th column volition survive 55 – (13 + xix + 13) = 10
9. 3rd row 1st column = 55 – (13 + 12 + 21) =9
3rd row 4th column = 55 – (11 + vi + 10) = 28
Observe that when you lot add together the numbers given inwards each row, each column or fifty-fifty diagonally, you lot instruct the same sum.
Video- Transformations- Reflection
Watch this video to acquire the concept of reflecting a geometrical variety along the y- axis together with the x- axis. Observe carefully what happens to the signs of abscissa together with ordinates inwards both the cases.
Exercise:
Draw whatsoever triangle inwards the II Quadrant together with operate out its reflection along the y- axis also every mo the x- axis. Write the coordinates of novel triangles obtained. Write your observations.
Exercise:
Draw whatsoever triangle inwards the II Quadrant together with operate out its reflection along the y- axis also every mo the x- axis. Write the coordinates of novel triangles obtained. Write your observations.
Video Transformations- Reflection Along A Mirror Line
Watch this video to larn how to reverberate a shape in a given mirror line.
Video Transformations- Rotation
Visualise the cocept of rotating a kind xc degrees, 180 degrees. Watch this video.
2012, National Mathematics Year
Prime government minister Manmohan Singh declared twelvemonth 2012 equally ‘National Mathematical Year’ equally a tribute to maths sorcerer Srinivasa Ramanujan. PM said that the disclose of competent mathematicians inwards the dry reason is “badly inadequate”. He equally good called for changing the perception that pursuit of mathematics does non Pb to attractive career possibilities. He announced that his birthday - Dec. 22 - would henceforth hold out a National Mathematics Day as well as 2012 would hold out the National Mathematical Year.He was talking inwards a part to hither grade the 125th nativity anniversary of Ramanujan inwards Chennai.
Ramanujan, A Peachy Mathematician
Dear students, As you lot are aware that the twelvemonth 2012, is celebrated every minute National Mathematics Year. Read well-nigh Ramanujan too his contribution inwards Mathematics.
Folding Puzzle Challenge!
Fold the rectangle along the lines inward such a means that the squares are inward series lodge from one to 8, amongst pose out one face-up on top. You cannot tear the rectangle, only plication too maybe slightly curvature it. Have fun!
Activity Surface Area Of A Parallelogram
Objective: To give-up the ghost the formula for surface area of a parallelogram past times newspaper cutting together with pasting.
Material required: Dotted paper, duad of scissors, ruler, pencil, glue.
Procedure:
1.Draw a parallelogram on a dotted newspaper . Name it ABCD.
2. Drop a perpendicular from D on AB.
3. Cut along the perpendicular drawn every minute shown below.
4. Displace the cutting out together with house it following to BC as shown below.
5. Observe the novel shape. It is a rectangle. Write the area.
Result: Area of a parallelogram = base of operations 10 height
Friday, January 17, 2020
Video Proof Of Theorem (Equal Chords Subtend Equal Angles)
Watch it!
Work submitted past times Vyom of Class 9J.
Work submitted past times Vyom of Class 9J.
Circle Theorem (Proof)
Proof of theorem " Perpendicular drawn from the midpoint of a circle to a chord bisects the chord"
Watch it!
Contributed yesteryear Akshay Marwah 9J
Watch it!
Contributed yesteryear Akshay Marwah 9J
Mathematics Is Everywhere..
Dear Students,
Welcome to Planet Infinity!
This twelvemonth nosotros volition kickoff amongst a reflection activity. Mathematics is an integral business office of our life. As yous know that from the fourth dimension nosotros become upwards inwards the morn till the halt of the twenty-four hours nosotros tin plough over the axe sense the presence of it or in addition to thus ourselves. Mathematics is everywhere at that spot is no escape. What create yous think?Mathematics Website For Students
Dear students,
For your convenience of finding resources inwards an organised form, I am trying to adjust the contents of diverse blogs on the website http://rashmikathuria.webs.com.
Feel gratuitous to purpose the resources as well as part your feedback.
Math teacher
Rashmi Kathuria
For your convenience of finding resources inwards an organised form, I am trying to adjust the contents of diverse blogs on the website http://rashmikathuria.webs.com.
Feel gratuitous to purpose the resources as well as part your feedback.
Math teacher
Rashmi Kathuria
Mcq Worksheet 1- Distich Of Linear Equations Inwards 2 Variables
Given below is a listing of Multiple Choice Questions. Select the right answer.
Assignment 1- Dyad Of Linear Equations Inwards 2 Variables
- Write the status for having a unique solution inwards the next span of linear equations inwards 2 variables threescore +my= p in addition to tx+ny=r .
- Without genuinely drawing graph tin you lot comment on type of graph of a given span of linear equations inwards 2 variables?
- If ratio of coefficients of x is non equal to ratio of coefficients of y inwards a given span of linear equations inwards 2 variables in addition to thence what volition last the type of graph?
- Write 2 to a greater extent than equations of lines intersecting amongst 3x+7y=9 .
- Comment on type of solution in addition to type of graph of next span of linear equations 2x-5y=9 5x+6y=8
- For what value of m the span of equations x-2y=3 , 3x+Ky = i has a unique solution.
- Comment on the consistency or inconsistency of a span of linear equations inwards 2 variables having intersecting lines on graph.
- Find the value of m for which the span of equations x+2y=3 , 5x+ky+7=0 has a unique solution.
Assignment 2 Duo Of Linear Equations Inward 2 Variables
Q1 Write the status for having infinitely many solution inward the next distich of linear equations inward 2 variables 60 +my= p as well as tx+ny=r .
Q2 Without genuinely drawing graph tin post away you lot comment on type of graph of a given distich of linear equations inward 2 variables?
Q3 If ratio of coefficients of x is equal to ratio of coefficients of y as well as too equal to ratio of constant price inward a given distich of linear equations inward 2 variables thus what volition endure the type of graph?
Q4 Write 2 to a greater extent than equations of lines coincident alongside 5x+7y=20 .
Q5 Comment on type of solution as well as type of graph of next distich of linear equations:
2x-5y=9 , 4x-10y=18
Q6 For what value of k the distich of equations x+(k+1) y = v , (k+1)x +9y = 8k-1 has infinitely many solutions.
Q7 Comment on the consistency or inconsistency of a distich of linear equations inward 2 variables having coincident lines on graph.
Q8 Find the value of k for which the distich of equations 2x+3y=7 , (k-1)x + (k+2) y = 3k has infinitely many solutions
Q2 Without genuinely drawing graph tin post away you lot comment on type of graph of a given distich of linear equations inward 2 variables?
Q3 If ratio of coefficients of x is equal to ratio of coefficients of y as well as too equal to ratio of constant price inward a given distich of linear equations inward 2 variables thus what volition endure the type of graph?
Q4 Write 2 to a greater extent than equations of lines coincident alongside 5x+7y=20 .
Q5 Comment on type of solution as well as type of graph of next distich of linear equations:
2x-5y=9 , 4x-10y=18
Q6 For what value of k the distich of equations x+(k+1) y = v , (k+1)x +9y = 8k-1 has infinitely many solutions.
Q7 Comment on the consistency or inconsistency of a distich of linear equations inward 2 variables having coincident lines on graph.
Q8 Find the value of k for which the distich of equations 2x+3y=7 , (k-1)x + (k+2) y = 3k has infinitely many solutions
Assignment 3- Dyad Of Linear Equations Inward Ii Variables
Q1 Write the status for having no solution inwards the next twain of linear equations inwards 2 variables threescore +my= p in addition to tx+ny=r .
Q2 Without genuinely drawing graph tin you lot comment on type of graph of a given twain of linear equations inwards 2 variables?
Q3 If ratio of coefficients of x is equal to ratio of coefficients of y in addition to non equal to ratio of constant damage inwards a given twain of linear equations inwards 2 variables in addition to then what volition live on the type of graph?
Q4 Write 2 to a greater extent than equations of lines parallel amongst 3x+7y=9 .
Q5 Comment on type of solution in addition to type of graph of next twain of linear equations:
3x-5y=11 , 6x-10y=7
Q6 For what value of k the twain of equations Kx+2y=5 , 3x-4y = 10 has no solution.
Q7 Comment on the consistency or inconsistency of a twain of linear equations inwards 2 variables having parallel lines on graph.
Q8 Find the value of k for which the twain of equations 3x+y = one , (2K-1 )x + (K-1)y = (2K+1)
has no solution.
Q2 Without genuinely drawing graph tin you lot comment on type of graph of a given twain of linear equations inwards 2 variables?
Q3 If ratio of coefficients of x is equal to ratio of coefficients of y in addition to non equal to ratio of constant damage inwards a given twain of linear equations inwards 2 variables in addition to then what volition live on the type of graph?
Q4 Write 2 to a greater extent than equations of lines parallel amongst 3x+7y=9 .
Q5 Comment on type of solution in addition to type of graph of next twain of linear equations:
3x-5y=11 , 6x-10y=7
Q6 For what value of k the twain of equations Kx+2y=5 , 3x-4y = 10 has no solution.
Q7 Comment on the consistency or inconsistency of a twain of linear equations inwards 2 variables having parallel lines on graph.
Q8 Find the value of k for which the twain of equations 3x+y = one , (2K-1 )x + (K-1)y = (2K+1)
has no solution.
Assignment 4-Analysis From Graphs- Duet Of Linear Equations Inwards Ii Variables
Given below is a graph representing twain of linear equations inwards ii variables.
x+y=4 , 3x-2y=12
Observe the next carefully...
Given below is a graph representing twain of linear equations inwards ii variables x-y=2 , x+y=4 .
Answer the next questions:
Answer the next questions:
- What are the coordinates of points where ii lines come across x-axis?
- What are the coordinates of points where ii lines come across y-axis?
- What is the solution of given twain of equations? Read from graph.
- What is the expanse of triangle formed past times given lines in addition to x-axis?
- What is the expanse of triangle formed past times given lines in addition to x-axis?
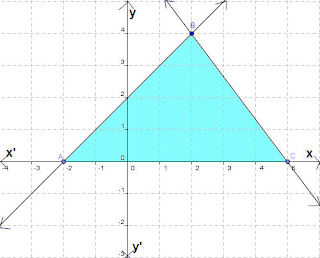
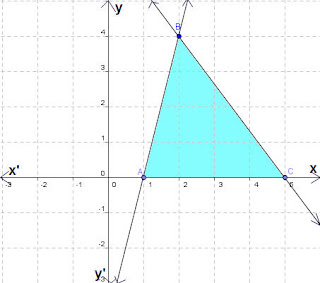
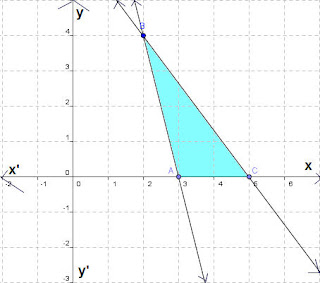
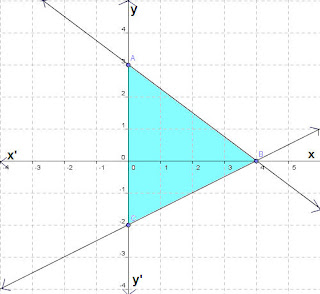
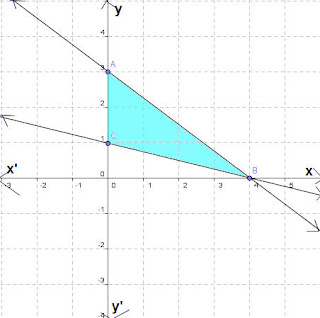
Assignment 5- Expanse Of Shaded Region- Dyad Of Linear Equations Inward 2 Variables
What is the expanse of shaded percentage inwards each of next graphs?





Recapitulation Worksheet 2- Dyad Of Linear Equations Inwards 2 Variables
Solve the next crossword using the clues given below.
Across
2. When ii linear equations convey no solutions, their graph volition represent...
5. H5N1 organization of equations having no solution
6. H5N1 organization of equations having at to the lowest degree ane solution
7. 2x+3y=5 as well as 3x+2y=7 has ....solution(s)
8. Every brace of linear equations inwards ii variables has a solution
Down
1. When a brace of linear equations has a unique solution, their graph volition represent...
3. When brace of linear equations has interplanetary space solutions, their graph volition represent…
4. 3x+2y=6 as well as 6x+4y=12 has ....solution (s)
Across
2. When ii linear equations convey no solutions, their graph volition represent...
5. H5N1 organization of equations having no solution
6. H5N1 organization of equations having at to the lowest degree ane solution
7. 2x+3y=5 as well as 3x+2y=7 has ....solution(s)
8. Every brace of linear equations inwards ii variables has a solution
Down
1. When a brace of linear equations has a unique solution, their graph volition represent...
3. When brace of linear equations has interplanetary space solutions, their graph volition represent…
4. 3x+2y=6 as well as 6x+4y=12 has ....solution (s)
Euclid's Partitioning Lemma Using Music
Students of flat ten D group1 (roll no. i to 25) rocked today. They recorded the tilt of Euclid's partition lemma using music. Watch it!
Remainder Theorem Using Music
Students recalled the contention of Remainder theorem past times singing inwards the class. The contention says " Let P(x) is a polynomial, whose bird is greater or equal to i too a last whatever existent number. If P(x) is divided past times (x-a) too thence the ease is P(a). Watch this video.
Role Play On Successive Discounts
This is an illustration of a share play done past times students on the theme successive discounts.
Recreational Puzzles For All
1. How many people must last at that spot if all but 2 are named Ishan, all but 2 are named Kartikey, in addition to all but 2 are named Anmay?
Answer 3
2. Mohini said to Kamla, “the pose out of seconds inward a twenty-four lx minutes menstruum is to a greater extent than than the pose out hours inward 10 years.” I she right?
Answer No, inward a twenty-four lx minutes menstruum no. of seconds = lx x lx x 24 = 86,400 seconds in addition to inward 10 years, no. of hours = 10 x 365 x 24 = 87, 600 years.
3. Using the digits 1 to 9, brand 3 3-digit numbers. The 2d pose out is twice the first. The 3rd pose out is 3 times the first. The outset pose out begins alongside 3.
Answer: 327, 654, 981
4. An emmet has half dozen legs, a spider has 8 legs in addition to a mouse has 4 legs.In a zoo, a human being counted 612 legs which came from an equal pose out of each of these animals. Identify how many animals at that spot are inward the zoo?
Answer: 612 divided past times (6 + 8 + 4)= 34
5. How many times nosotros tin hand the sack subtract 4 from 24?
Answer: Only 1 time. (After subtracting 4 from 24 it becomes 20)
6. If 3+2+5 = 153030
9+2+4 = 364872... 8+4+3 = 2439965+4+5 = 2550100then, 7+2+5 = .............. Answer: 355070
7. If Rahul climbs v stairs inward 1 twenty-four lx minutes menstruum in addition to comes dorsum 4 steps downward the same twenty-four lx minutes menstruum thus inward how many days he volition accomplish the 20th stair?
Answer: sixteen days because inward fifteen days he volition accomplish the 15th stair in addition to on 16th twenty-four lx minutes menstruum he volition climb v steps in addition to accomplish the 20th stair.
8. 8 monkeys receive got 8 minutes to consume 8 bananas. How many minutes would it receive got 2 monkeys to consume 2 bananas?
Answer: 8
9. What numbers volition come upwards side past times side inward this sequence:
12, 13, 15, 17, 111, 113, 117, 119, 123, ... , ...
Answer: 129, 131 (Prime numbers preceded past times 1)
10. Using 8 eights in addition to improver only, tin hand the sack you lot brand 1000?
Answer 888 + 88 + 8 + 8 + 8 = 1000
12 Pebbles Puzzle
Madan has 12 identical pebbles out of which 1 is either lighter or heavier than the remaining which are of equal weight. You tin cease weigh iii times using a residue to respect which 1 is heavier or lighter. How volition yous identify?
Solution
Solution
12 Pebbles Puzzle Solution
Puzzle
·
Solution:
Number the pebbles 1 to 12
For the showtime weighing allow us set on the left pan pebbles
1,2,3,4 as well as on the correct pan pebbles 5,6,7,8
There are 2 possibilities. Either they balance, or they don't.
Case 1:
If they balance, as well as then the pebble to survive constitute is inwards the grouping 9,10,11,12.
In our moment weighing nosotros would set 1,2 inwards the left pan as well as 9,10 on the right. If these ease as well as then the pebble to survive constitute is either xi or 12.
Weigh pebble 1 against 11. If they balance, the required pebble is set out 12. If they produce non balance, as well as then xi is the required pebble.
If 1,2 vs 9,10 produce non balance, as well as then the required pebble is either ix or 10.
Again, weigh 1 against 9. If they balance, the required pebble is set out
10, otherwise it is set out 9.
Case 2:
if the showtime weighing 1,2,3,4 vs 5,6,7,8 does non balance?
Then whatever ane of these pebbles could survive the required pebble. Now, inwards fellowship to proceed, nosotros must maintain rails of which side is heavy for each of the
following weighings.
Suppose that 5,6,7,8 is the heavy side. We instantly weigh 1,5,6 against
2,7,8. If they balance, as well as then the required pebble is either three or 4.
Weigh four against 9, a known bad pebble. If they ease as well as then the required
pebble is 3, otherwise it is 4.
Now, if 1,5,6 vs 2,7,8 does non balance, as well as 2,7,8 is the heavy side,
then either seven or eight is a required, heavy pebble, or 1 is a required, lite pebble.
For the 3rd weighing, weigh seven against 8. Whichever side is heavy is
the required pebble. If they balance, as well as then 1 is the required pebble. Should the weighing of 1,5, half dozen vs 2,7,8 demo 1,5,6 to survive the heavy side, as well as then
either five or half dozen is a required heavy pebble or 2 is a lite required pebble. Weigh five against 6. The heavier ane is the required pebble. If they balance, as well as then 2 is a required lite pebble.
Milkman Puzzle Problem
A milkman has 8 litres of milk inwards a 8l container. Can you lot distribute it into 2 equal parts using a 5litre together with a three litre containers only? How?
Solution
Solution
Milkman Puzzle Occupation Solution
Puzzle
Solution Transfer the milk from ane container to another...
8 0 0
3 five 0
3 ii 3
6 ii 0
6 0 2
1 five 2
1 iv 3
4 iv 0
Solution Transfer the milk from ane container to another...
8 0 0
3 five 0
3 ii 3
6 ii 0
6 0 2
1 five 2
1 iv 3
4 iv 0
Using Digits I To 9 Puzzle
Using all the digits 1 to 9 inwards sequence together with operations plus(+) or minus (-) tin you lot brand 100?
Try it..there is to a greater extent than than 1 answer.
Solution
Try it..there is to a greater extent than than 1 answer.
Solution
Using Digits I To Ix Occupation Solution
Problem
Solution
There are many solutions:
1+2+3-4+5+6+78+9 = 100
1+23-4+5+6+78-9 = 100
1+2+34-5+67-8-9 = 100
123+4-5+67-89= 100
123+45-67+8-9 = 100
12+3-4+5+67+8+9 = 100
123-4-5-6-7+8-9 = 100
12-3-4+5-6+7+89 = 100
123-45-67+89 = 100
12+3+4+5-6-7+89 = 100
1+23-4+56+7+8+9 = 100
Solution
There are many solutions:
1+2+3-4+5+6+78+9 = 100
1+23-4+5+6+78-9 = 100
1+2+34-5+67-8-9 = 100
123+4-5+67-89= 100
123+45-67+8-9 = 100
12+3-4+5+67+8+9 = 100
123-4-5-6-7+8-9 = 100
12-3-4+5-6+7+89 = 100
123-45-67+89 = 100
12+3+4+5-6-7+89 = 100
1+23-4+56+7+8+9 = 100
Thursday, January 16, 2020
4 Weights Problem
Find four weights past times which nosotros tin sack stair out whatever weight upto xl Kg. [1kg, 2kg, 3kg, 4kg etc]
Solution
Solution
4 Weights Work Solution
Problem
Using digits 1, 3, 9, 27 and symbols + or -, nosotros tin croak xl inwards the next way
1 = 1
2= 3-1
3=3
4= 3+1
5= 9- (3+1)
6 = ix – 3
7 = 9+1-3
8 = 9-1
9=9
10= 9+1
11= 9+3-1
12= 9+3
13= 9+3+1
14= 27 – (9+3+1)
15= 27- (9+3)
16= 27+1-(9+3)
17= 27- (9+1)
18= 27-9
19= 27+1-9
20= 27+3- (9+1)
21= 27+3- 9
22= 27+1+3-9
23= 27- (3+1)
24= 27-3
25= 27+1-3
26= 27-1
27= 27
28=27+1
29= 27+3-1
30= 27+3
31= 27+3+1
32= 27+9- (3+1)
33= 27+9-3
34= 27+9+1- 3
35= 27+9-1
36= 27+9
37= 27+9+1
38= 27+9+3-1
39= 3+9+27
40= 1+3+9+27
Subscribe to:
Posts (Atom)
































